The Gilbreath Principle
By Matt Baker - Wednesday, October 14, 2020
In this post I’ll be talking about the Gilbreath Principle, which Max Maven has memorably described as “a thing of terrifying beauty”. To learn what the Gilbreath Principle is all about, grab a deck of cards and take out three red cards and three black ones. No really: go get a deck of cards. I’ll wait.

Place three cards face-down to your left: a black card, then a red card, and then a black card. Place the other three cards face-down to your right, but in the opposite order: a red card, then a black card, then a red one. Now take the top card from either pile and place it face-down in front of you. Do the same thing again, placing another card from the top of either pile face-down on top of the previous one. Repeat this same procedure with the rest of the cards; you now have a pile consisting of six face-down cards in front of you. Take a look at the top two cards.
ONE OF THESE CARDS WILL BE RED AND THE OTHER WILL BE BLACK.
Same for the second pair, and the third pair as well. Each of the three pairs, thanks to this marriage of mathematics and magic, will have exactly one red card and one black card.
If you want to know why this works, repeat the same experiment again but this time with the cards face-up. I think you’ll quickly see what’s going on. For the sake of argument, choose the black card first. What do you see in front of you now? Two piles, each with a red card on top! You can choose which pile to pick from, but no matter how you slice it the next card is going to be red. Take one of those red cards and place it on top of the black card that you previously selected.
The situation at this point mimics the initial one: there are two piles, each with a different color on top, and the cards in each pile alternate colors. So the same thing will happen in the second round that happened in the first! Whichever choice you make for the next card, the one after that will always be of the opposite color. And then you’ll be left with two more cards, one of each color. This, in essence, is why the Gilbreath Principle works.
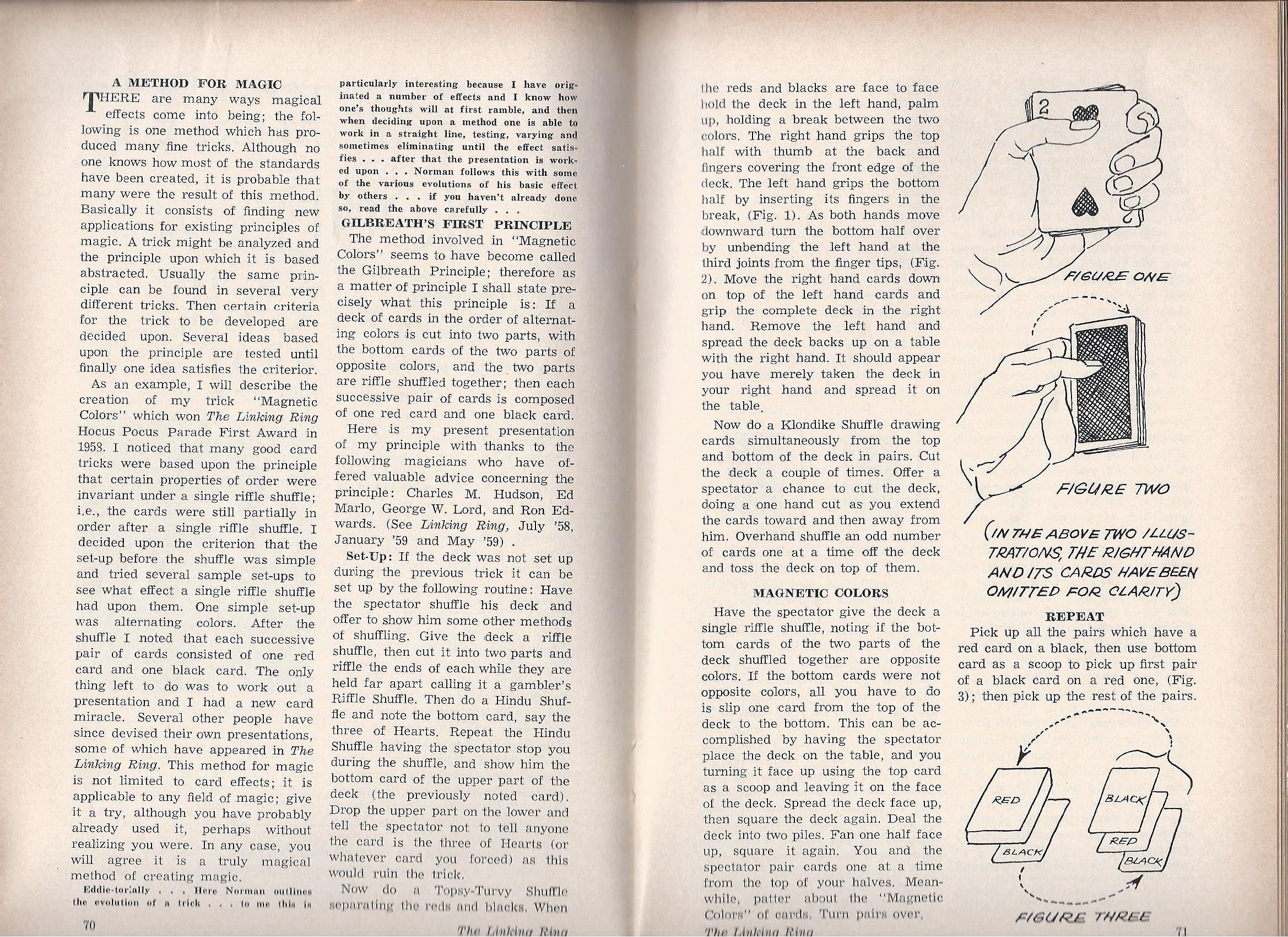
By itself, what we’ve just described is not particularly magical (especially when done face-up!). But the applications to card magic are immense. For example, here’s a parlor-style demonstration that I like to perform. In terms of method, it’s identical to Norman Gilbreath’s “Magnetic Colors” (from the 1958 Linking Ring column where Gilbreath first published his ideas on the principle). However, I think that some readers will find value in the presentation which follows.
Start with a full deck (or perhaps 40 cards, if you want things to go quicker) in which the cards secretly alternate red and black. I like to use jumbo cards for this demonstration, and in fact I typically use cheap thin jumbo cards from the dollar store because they’re much easier to riffle shuffle than regular stock. Bring up a volunteer from the audience who likes to gamble. Say that you’re going to play a game, and will be wagering on the outcome, but you need him to agree first to follow the rules. When he agrees, you proceed to explain the rules he’s just agreed to (this is usually a funny moment).
Tell the spectator that you will show the cards one pair at a time. If they’re both red, the spectator wins. If they're both black, the spectator also wins. You, the magician, only win if the cards are different colors. Since the spectator has two ways to win and you only have one, each time the spectator wins you will give him a dollar, but each time you win the spectator will give you two dollars. Someone in the audience will likely express suspicion that this is not in fact a fair bet. Remind the volunteer that he already agreed to play by your rules, so you don’t have to do this, but since you’re a nice guy you’ll make it even odds. Point out that you also don’t have to let the spectator cut the cards, because that wasn’t part of the agreed-upon rules, but since you’re such a nice guy you’ll let him do it anyway. Have the spectator cut and complete.
Say that you’re in fact SUCH a nice guy that, against your better instincts, you’ll even let the spectator shuffle the cards. Cut the deck roughly in half and instruct the spectator to riffle shuffle the two halves together. Quickly deal the cards into two piles, and while you’re doing this designate someone in the audience to keep track of who has won how much money. You now proceed to show the top card from each pile, one pair at a time. Amazingly, you end up winning all of the money with a 100% sweep of the head-to-head contest.
The trick is self-working, thanks to the Gilbreath Principle. All you need to do is ensure, as you riffle through the pack in order to cut the cards into two piles for the spectator to shuffle, that the bottom cards of the two piles have opposite colors. That’s it, that’s the only thing you have to do in the entire trick other than be your own hilarious self.
In case it isn’t already obvious, a riffle shuffle of two face-down piles accomplishes the same thing as choosing the top card from either of two face-up piles (as in our previous “hands-on” demonstration). Indeed, when you riffle shuffle two halves together, each time one of your thumbs releases a card you are effectively just selecting the bottom card from one of the two piles.
Now it’s time for a confession: so far, we’ve only been talking about Gilbreath’s FIRST Principle. There is also a SECOND Gilbreath Principle, which generalizes the first. We’ll get back to that in a little bit… However, I’d like to skip ahead all the way to what Diaconis and Graham refer to as the “Ultimate Gilbreath Principle”.
Suppose we have a deck of N cards (you can imagine that N=52 if you like, but the principle applies to a deck of any size). Each card has a “stack number”, with the top card of the face-down deck having stack number 1, the next card stack number 2, etc., all the way down to the final card which has stack number N.
Pick up the face-down deck and deal as many cards as you like into a face-down pile on the table. Call the pile on the table Pile A and the cards left in your hand Pile B. Place Pile B next to Pile A and riffle shuffle the two packets together. (This does NOT have to be a perfect shuffle, any kind of riffle shuffle will do.)
Now lift off ANY number of cards from the top of the shuffled deck on the table. Believe it or not, the following will always hold true of the cards in your hand:
The Ultimate Gilbreath Principle: The cards which you lifted off can be rearranged so that their stack numbers are consecutive.
For example, suppose we have N=10 cards numbered 1,2,3,…,10. (If you want to follow along, take out an Ace through 10 and arrange the in numerical order.) Deal down four cards to form pile A on the table (which is now in the order 4-3-2-1) and pile B in your hand (which is in the order 5-6-7-8-9-10). Now riffle shuffle piles A and B together.
One possible arrangement of the deck at this point is 5-6-4-7-8-3-9-2-10-1. Observe, for example, that:
- If you lift off the top 3 cards (5-6-4), they can be rearranged to form the consecutive block 4-5-6.
- If you lift off the top 5 cards (5-6-4-7-8), they can be rearranged to form the consecutive block 4-5-6-7-8, etc.
Another possible arrangement of the deck (if it was shuffled very badly) is 5-6-7-8-9-4-10-3-2-1.
In this case:
If you lift off the top 6 cards (5-6-7-8-9-4), they can be rearranged to form the consecutive block 4-5-6-7-8-9.
If you lift off the top 8 cards (5-6-7-8-9-4-10-3), they can be rearranged to form the consecutive block 3-4-5-6-7-8-9-10, etc.
I hope the statement of the Ultimate Gilbreath Principle is now clear. But WHY does it work?
As before, let’s try it with the cards FACE UP to give you an idea. Put the cards in A-10 order from the face of the deck and deal four cards face up into a pile (Pile A) on the table. Place the cards in your hand (Pile B) next to Pile A. Let’s make a new “shuffled” packet by choosing the top card from either pile A (the top card is currently a 4) or pile B (currently a 5). If you chose the 4 from Pile A, the new top cards are a 3 in Pile A and a 5 in Pile B. Either way, the next card you choose will form a consecutive block with the 4 you just picked! On the other hand, if you instead chose the 5 from Pile B, the new top cards are a 4 in Pile A and a 6 in Pile B. Again, either way, the next card you choose will form a consecutive block with the 5. This pattern of choices keeps recurring as you choose more and more cards, just like in the original Gilbreath Principle.
But wait, what does this have to do with red-black pairs and Gilbreath’s First Principle? Well, suppose you begin with a stack which alternates R-B-R-B-R-B, as in the beginning of this article. Cut the deck into two piles of three cards each, with Pile A alternating R-B-R and Pile B alternating B-R-B. Reverse counting Pile A does not change the fact that the order is R-B-R, so we can ignore that step here. Now shuffle the two piles together. By the Ultimate Gilbreath Principle, the first two cards are consecutive in the original stack order, which implies that one is red and one is black (there are no consecutive R-R or B-B pairs in the initial alternating stack). Similarly, the UGP shows that out of the first FOUR cards in our shuffled packet, two are red and two are black. In particular, since we already know that there’s one red and one black card among the first pair, it follows that the second pair of cards also contains one red and one black. We can deduce that the third pair consists of one red and one black as well. A generalization of this argument shows that the UGP implies the original version of the Gilbreath Principle.
In a similar way, the Ultimate Gilbreath Principle also implies Gilbreath’s Second Principle, which says (in the words of Charles Hudson):
“When a repeating series of cards is riffle shuffled into itself, with one of the packets to be
shuffled in reverse order to the other, the contents of each group in the series do not change -
they are only disordered.”
For example, if you have a deck in CHaSeD order and follow the reverse count / riffle shuffle procedure above, the first four cards will contain exactly one card of each suit, and similarly for the next four, and the next four after that…
And now for a fun application. There are two routines in my book The Buena Vista Shuffle Club which make use of the Gilbreath Principle: “Card College”, which combines the Ultimate Gilbreath Principle with a memorized deck, and “Gilbreath Poker”, an interactive self-working poker demonstration inspired by a routine in Diaconis and Graham’s book Magical Mathematics. “Card College” is too elaborate to explain here, but for the reader’s convenience I will now explain a streamlined version of my “Gilbreath Poker” routine.
Effect: The magician offers to teach a volunteer, Joanna, how to cheat at poker, saying it’s all about skillful shuffling and dealing. The magician explains that only 25 cards will be needed, and he quickly counts 25 cards to the table, splits the tabled pile roughly in half, and hands the two piles to Joanna. The remainder of the deck is put away. The magician asks Joanna to riffle-shuffle the two packets together “as skillfully as possible”. The audience is encouraged to study Joanna’s technique, which the magician compliments. The magician now instructs Joanna to deal the cards into 5 poker hands of 5 cards each, with the goal of dealing the player to her left — “the mark” — an excellent hand, but to deal herself an even BETTER hand. The mark’s hand and dealer’s hand are turned over, but nothing particularly exceptional has happened. After a brief puzzled look, the magician exclaims, “I know what happened — we forgot to cut the cards! You always have to cut the cards in a game of poker.” Joanna reassembles the five poker hands (in whatever order she likes), gives the cards a cut, and then deals out another round. This time, Joanna is shown to have dealt a full house to the player on her left and a straight flush to herself!
Method: There is a 25 card setup for the trick. Begin with the four Aces on top, followed by three red indifferent cards, the Q-J-10-9-8 of Spades, the four Kings, four red indifferent cards, and 3-4-5-6-7 of Spades. As you count 25 cards face down, in-jog the 13th card slightly. Separate the cards at the in-jog (with the in-jogged card being the bottom card of the right-hand portion) and place the two face-down piles in front of the spectator to shuffle. All you need to do is guide Joanna through a Jay Ose False Triple Cut (see below if you’re not familiar with this), and — believe it or not — the rest of trick is self-working. (Occasionally the “mark” will get four of a kind instead of a full house.) Note that, in addition to the Gilbreath Principle, we are making use of the Gardner-Marlo Poker Deal principle.
The Jay Ose Triple Cut works as follows. Have the spectator lift off approximately a third of the cards in their hand and place them to their left. They then cut off about half of the remaining cards and place them to the right of the previous packet, then place the remaining cards to the right of the other two packets. Have them reassemble the packets from left to right, placing each one on top of the previous combined packet. This results in a rather deceptive false cut. The idea of having the spectator perform this false cut on them self is due to Paul Harris (see Chad Long’s “The Poker Lesson” from The Art of Astonishment, Book 3).
There are literally hundreds of other Gilbreath Principle-based routines listed on Denis Behr’s Conjuring Archive. Here are some personal favorites:
- A deliciously devious application of the First Gilbreath Principle is Asi Wind’s “True Colors”, from his book Repertoire. Hector Chadwick has a similar routine called “Reds and Blacks” in his book The Mental Mysteries of Hector Chadwick.
- Max Maven has a great deal of work on the principle, much of it still unpublished, including “Zenvelopes” and “The Mockingbird”, from his “Videomind” series, and “Word Row”, from his book Redivider.
- Woody Aragon has a number of clever applications of the Gilbreath Principle in A Book in English, including “Si Fry” and “Grandpa’s Poker”.
- Some other excellent Gilbreath Principle-based routines to check out include David Williamson’s “Aunt Mary’s Terrible Secret”, Sal Piacente’s “Memory Opener”, Jack Carpenter’s “Ultimate Hold ‘Em Demonstration”, and Tomas Blomberg’s “Photo Vibrations”.
And finally, a few historical notes and credits:
- According to https://www.conjuringcredits.com/doku.php?id=cards:gilbreath_principle: “There were several “proto-Gilbreath” (as Max Maven calls them) tricks that predate Gilbreath's own work, where the principle was there, but just not fully understood at the time. This includes work by Charles Jordan and J. Russell Duck.”
- Norman Gilbreath did not publish the second version of his namesake principle until 1966, some eight years after the first. According to Charles Hudson, George Lord independently discovered Gilbreath’s Second Principle about two months after the publication of Normal Gilbreath’s “Magnetic Colors” but did not publish it.
- The streamlined “Gilbreath Poker” routine explained above shares several ingredients in common with an effect from Steve Mayhew’s booklet Angels May Shuffle But the Devil Still Deals entitled “Ultimate Gardner-Marlo”.
- Thanks to Persi Diaconis for sharing his ideas on the slimmed down version of “Gilbreath Poker” presented here. Stefan Gruber suggests replacing the line “I know what happened — we forgot to cut the cards! You always have to cut the cards in a game of poker” with “I think perhaps the stakes are too low. We need to place a bet!” and putting some money on the table.
- For theoretically inclined readers, it may be worth remarking that the “Ultimate Gilbreath Principle” can be used, in a precise mathematical sense, to characterize riffle shuffles. See the Diaconis-Graham book for further details and a proof.
Back to blog homepage
Similar posts on the blog:

